Spurpunkte

Die Schnittpunkte der Geraden mit den Koordinatenebenen $E_{xy}$, $E_{xz}$, $E_{yz}$ nennt man Spurpunkte.

!

Merke

Eine Gerade kann 1, 2, 3 oder unendlich viele Spurpunkte haben.

i

Vorgehensweise

Entsprechende Koordinate gleich Null setzen und $r$ berechnen
$r$ in die Geradengleichung einsetzen, um Spurpunkt zu erhalten

i

Tipp

Bei den Ebenen ist immer die Koordinate Null, die nicht im Namen vorkommt.

$E_{xy}: z=0$
$E_{xz}: y=0$
$E_{yz}: x=0$

Beispiel

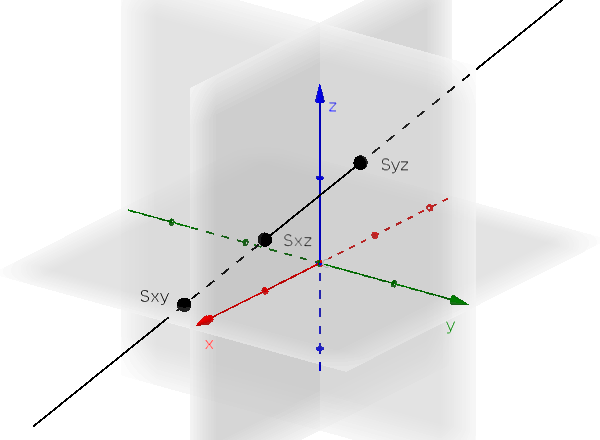
Berechne den Spurpunkt der Geraden $g$ mit der xy-Ebene.

$\text{g: } \vec{x} = \begin{pmatrix} 1 \\ 2 \\ 3 \end{pmatrix} + r \cdot \begin{pmatrix} 4 \\ 5 \\ 6 \end{pmatrix}$

$r$ berechnen
Da es sich um die Ebene $E_{xy}$ handelt, setzen wir z gleich 0.
$\begin{pmatrix} x \\ y \\ 0 \end{pmatrix} = \begin{pmatrix} 1 \\ 2 \\ 3 \end{pmatrix} + r \cdot \begin{pmatrix} 4 \\ 5 \\ 6 \end{pmatrix}$

Die Zeile mit nur einer Variablen (hier die dritte) wird nach $r$ umgestellt.

$0=3+6r\quad|-3$
$-3=6r\quad|:6$
$r=-0,5$
Spurpunkt bestimmen

$\text{g: } \vec{x} = \begin{pmatrix} 1 \\ 2 \\ 3 \end{pmatrix} + r \cdot \begin{pmatrix} 4 \\ 5 \\ 6 \end{pmatrix}$

Das berechnete $r=-0,5$ wird in die Geradengleichung eingesetzt.

$\begin{pmatrix} 1 \\ 2 \\ 3 \end{pmatrix} + (-0,5) \cdot \begin{pmatrix} 4 \\ 5 \\ 6 \end{pmatrix}$ $=\begin{pmatrix} -1 \\ -0,5 \\ 0 \end{pmatrix}$

Der Spurpunkt mit der xy-Ebene ist $S_{xy}(-1|-0,5|0)$